Here, you're going to learn some calculations and properties related to geodesic domes. For example, in a small time, you'll be able to calculate the number of faces, vertices ans sides of any geodesic dome. However, you must know the three parameters of a geodesic dome : N, a and b (here are definitions of parameters).
You also have to know if the geodesic dome is a normal or a dual geodesic dome, because calculations are different (click if you don't remind what's a regular or a dual geodesic dome).I Calculations
We'll call F the number of faces, V the number of vertices and S the number of sides. First, we must calculate 2 other variables, which we called x and y. The calculations of x and y are :
In a regular geodesic dome :
The calculations of S, V and F are :
A regular geodesic dome has two types of vertices : many vertices are vertices of 6 faces at the same time (called V6), but some others are vertices of N faces (called VN). the calculations of V6 and VN are :


In a dual geodesic dome :
Here are the calculations of F, S and V :
A dual geodesic dome has two types of faces : many faces have 6 sides (called F6, these are hexagons), but some others have N sides (called FN, so triangles when N=3, squares when N=4 and pentagons when N=5). The calculations of F6 and FN are :
You can see formulas are identical in a regular or in a dual geodesic dome, but what you calculate is different (for example, we have the same calculations between the number of faces in a regular geodesic dome and the number of vertices in the dual geodesic dome). Don't forget that the construction of a dual geodesic dome is very close to that of the regular !
II Properties
Here are properties of 3 particular geodesic domes :
- If a=1 and b=0, the geodesic dome is the beginning solid. In fact, you cut all sides in a+b=1, so the solid remains the same.
- We can think that faces of geodesic domes are equilateral triangles, but it's wrong, except when a=b=1.
- If a=b or b=0, the geodesic dome has the same properties as its beginning polyhedron. For example, it has 15 planes of symmetry which cross 2 opposite sides. Otherwise, it doesn't have any plane of symmetry.
III Application in the Biosphere
As the Biosphere is a geodesic dome, we can use these calculations.
As you know, the three parameters (N, a and b) equal 5, 16 and 0 in the Biosphere. Moreover, these are "two" geodesic domes in the Biosphere, the normal and the dual. So, we're going to make all these calculations. Our calculations will be made as if the geodesic dome is "complete".
First, we must calculate x and y. So :
The normal geodesic dome :
The most visible geodesic dome in the Biosphere is the "normal", so we'll begin with this one.
I agree that it seems too big ! You probably think that it's not possible, but I answer that we can't imagine just looking at the Biosphere the number of faces. Our eyes easily deceive us !
2550 + 12 = 2562, so we find the total number of vertices : it's correct.
The dual geodesic dome :
As I said before, the results are the same, but not for the same thing.
Then, you can see that mathematics are once again omnipresent in architecture : here, in an architecture like the geodesic dome and the Biosphere, the area of algebra dominates too.

.gif)
.gif)
.gif)
.gif)



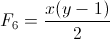


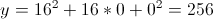





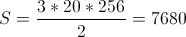




No comments:
Post a Comment