Parameters of a Geodesic Dome

 So, we're going to begin with the construction of a geodesic dome. For that, you have to know and understand the three parameters of a geodesic dome. This parameters were discovered by Richard Buckminster Fuller during his experiences in 1948-1949. He also showed that geodesic domes are ideal for gorgeous architectures.

The first parameter is the number of faces which have a vertex in common, called N.
 For example, in a tetrahedron N is equal to three, in an octahedron to four and in an icosahedron to five.

 Tetrahedron (N = 3)

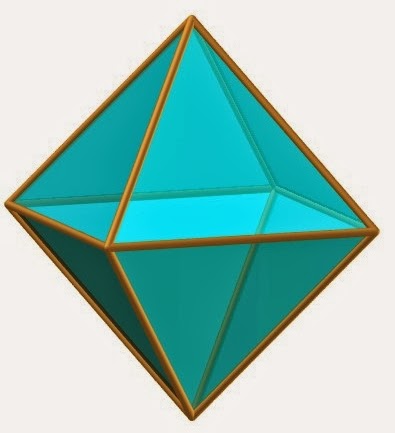Octahedron (N = 4)

Icosahedron (N = 5)

 A step in the construction of a geodesic dome consists of the separation of each side of a triangle in equal segments. If we take the triangle with a vertex at the top, we rely it with a point of the opposite side which have a equal segments on its left and b equal segments on its right. b is greater or equal to 0. a is greater than 0 and greater or equal to b.

a and b are the two other parameters of a geodesic dome and a+b is called frequency.

Example :  a = 3 and b = 2

Note :

• When b = 0, the geodesic dome belongs to class I and is called "triacon". 
• When a = b, the geodesic dome belongs to class II and is called "alternate". 
• The other cases belongs to class III. 

In the Biosphere, N = 5, a = 16 and b = 0. It belongs to the "triacon" class.